FLASH FRIDAY is a weekly content series looking at the past, present and future of capital markets trading and technology. FLASH FRIDAY is sponsored by Instinet, a Nomura company.
This year marks the 50th anniversary of Fischer Black and Myron Scholes publishing what has become known as the Black-Scholes formula, as well as Robert Merton publishing a separate paper providing a mathematical analysis of their model and extending it. In 1973 the three young Ph.D. students probably had little idea that their formula would lead to the development of a multi-trillion dollar derivatives industry. The equation has been described as both the formula that developed Wall Street and as the formula that caused the 2008 financial crisis.
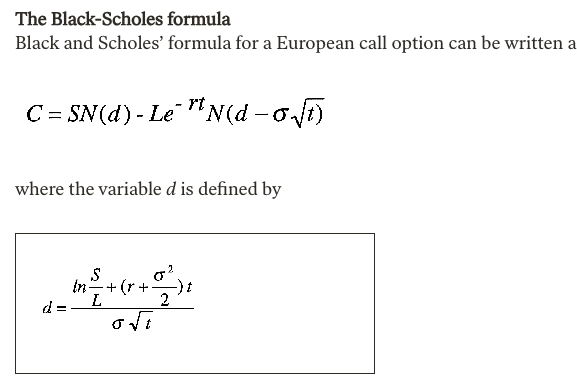
The papers described how to calculate the price of an option, a contract which gives the holder the right, but not the obligation, to buy or sell an underlying financial asset at a set price on a predetermined future date. The holder of the contract can decide whether or not to exercise the option based on the market price of the financial asset on that date, compared to the predetermined price of the asset in the contract. The derivative amounts to a form of insurance for both parties by providing a guaranteed market at a guaranteed price, independent of shifts in the market value, in return for a premium.
Peter Tankov, professor of quantitative finance at ENSAE (IP Paris), explained that the equation provides a strategy for hedging the option. He wrote in a blog: “A trader selling an option at the price given by the Black-Scholes formula can immediately put in place a strategy, allowing to minimize, if not completely eliminate, the risk associated to this position. Prior to Black and Scholes, such dynamic hedging strategies could not be computed in a systematic way, which slowed down the development of derivatives markets.”
In 1997 the Nobel prize in Economics was awarded to Merton and Scholes for their collaboration with the late Black, who had died in his mid-fifties in 1995. The Nobel committee said a new method to determine the value of derivatives stood out among the foremost contributions to economic sciences over the previous 25 years.
In the presentation speech for the award Professor Bertil Näslund of the Royal Swedish Academy of Sciences said options contracts go back to ancient Greece and that they were actively traded in Amsterdam in the seventeenth century. However, until the end of the 1960 there was no fully acceptable method of evaluating and pricing option contracts.
Näslund continued that soon after Black, Merton and Scholes published their landmark papers the formula was applied on a new options exchange in Chicago and it became used daily by thousands of agents on markets all over the world.
“More important than the formula itself, however, was the method that this year’s Laureates used to derive it,” Näslund added. “In one stroke they solved the problem which had been an obstacle in the pricing of all kinds of options, that is: what risk premium should be used in the evaluation.”
Their answer was that no risk premium was needed, and in combination with advances in technology, this generated an explosive growth of new financial products and markets.
Scholes told the BBC the Chicago Board Options Exchange started to trade call options on 16 stocks about a month after his article was published in 1973.
He and Merton subsequently became partners of hedge fund, Long-Term Capital Management (LTCM), that based its trading on mathematical principles such as the Black-Scholes equation. However, in 1998 the fund lost $4.6bn in under four months after the Russia crisis and the Federal Reserve had to organise a bail out by a group of banks.
However, Scholes argued the hedge fund’s collapse had nothing to do with equations or models. He told the BBC: “I was not running the firm, let me be very clear about that. There was not an ability to withstand the shock that occurred in the market in the summer and fall of late 1998. So it was just a matter of risk-taking.”
The question of modelling and risk management resurfaced in 2008 after the collapse of Bear Stearns and Lehman Brothers amidst the financial crisis.

Ian Stewart, emeritus professor of mathematics at the University of Warwick included the Black-Scholes formula in his book Seventeen Equations That Changed the World, calling it the “Midas Formula.” He asked: “How did the biggest financial train wreck in human history come about? Arguably, one contributor was a mathematical equation.”
Stewart explained that the equation expresses the rate of change of the price of the derivative, with respect to time, as a linear combination of the price of the derivative itself, how fast that changes relative to the underlying asset price and how that change accelerates. However, it assumes perfect information, perfect rationality, market equilibrium and the law of supply and demand.
“If the assumptions behind the model ceased to hold, it was no longer wise to use it,” wrote Stewart. “But as time passed and confidence grew, many bankers and traders forgot that; they used the equation as a kind of talisman, a bit of mathematical magic that protected them against criticism.”
He argued this was demonstrated by the collapse of LTCM as soon as reality ceased to obey the assumptions of the model. Despite this warning, credit default swaps and collateralized debt obligations, derivatives that were implicated in the 2008 financial crisis, were priced using descendants of the Black–Scholes equation. However, Stewart also rightfully concludes that an equation is just a tool that has to be used by someone who knows how to use it properly and for the right purpose.
“The Black–Scholes equation changed the world by creating a booming quadrillion-dollar industry; its generalisations, used unintelligently by a small coterie of bankers, changed the world again by contributing to a multi trillion-dollar financial crash whose ever more malign effects, now extending to entire national economics, are still being felt worldwide,” wrote Stewart.
Let’s hope that the equation is used more intelligently over the next 50 years.








